On Rejection of Scales
Musicology and music theory books didn’t use the term microtonality until the beginning of 20th or end of 19th century, to clarify what was previously known as Xenharmonic music, sometimes quarter-tone music or similar names. Basically addressing music that involves intervals smaller than semitones of Western equal temperament music. And while the general belief may be that what is commonly referred to as microtonal music (vaguely music that applies intervals other/smaller than semitones) is an equally new style or trend of music making, the historical record of the actuality of the music shows otherwise.
In fact the need for having a term to describe music with intervals other than equal temperament came to necessity only after the equal temperament systems were established and became the status quo, as a keyword to point deviation from the established system. In this regard, the music had always been so called microtonal.
The current microtonal music, as is commonly accepted in musical/microtonal music communities, is chiefly used in regard to a scale of musical intervals less (or other) than 12-tone equal temperament. Scale here meaning divisions of an interval, universally agreed to be an octave, into certain steps. But the scale(s) weren’t readily available or existing for a long time.
Background
We know that earliest documentation of musical systems/theories, at least those that have reached us, have been done by scientists. After all study of music was part of academia’s syllabi and a branch of science, in almost all ancient civilizations. Looking at history from Greek antiquity till today it has not usually been the artist who investigated and theoretically studied his artwork, probably for a good reason too. As much as these studies have been crucial and useful, they’ve proven to be somewhat confusing or in some cases even controversial.[1]
The supposed separation of practice and theory, have eventually benefitted both. This approach greatly affected the conception, understanding and the framework of art and philosophy.
In the modern days, with new technologies and accessibility of books and written documents, those older documentation became source of study for artists. Thus the way we understand and perceive the music is greatly shaped by the ways mathematicians/scientists or theorists had defined, documented and systemized it.
Iconographical evidence
The oldest traces of music, actual evidence, are found from archeological or indeed archeoacoustical exploration. These evidences are usually administrative documentation concerning musicians and/or some indications of instruments that produce sound (including primitive percussive instruments). Functionality of these sounds could be varied from ceremonial to sacred or even communication. In best cases an actual instrument of historic value is the closest and best information to the approximate actuality of music.
We can easily suppose that the vocal music has predated the instrumental music. After all, before inventing any tools mankind was born with ability of making sound with their voice. In this view the music, on its most basic definition, predates the language, or else has been one of founding elements of creating languages.
Theoretically the music could be traced back prior to Paleolithic era, as early as when stone tools were used. The earliest instruments of making music (sound) have been simple percussive tools. The oldest found pitched instrument is the bone flute dubbed as “Neanderthal flute” from 43000 years ago (figure 1).
The discovery of these 35000 years old flutes at Hohle Fels appears to indicate a rather sophisticated musical tradition. Of the three flutes found, the largest one has 5 holes and can produce what resembles a pentatonic span of pitches. It is noteworthy that there are small, carved marks near the holes that are presumed to be related to the measurement for placing the holes.


Figure 1. The flutes found near Hohle Fels caves. Photo Credit: Jensen / University of Tubingen
Theory and practice
In this example, a replica recreation of one of the Hohlen Fels is being played. The player, realizes a modern pentatonic scale from the hole placements, and goes on to play a contemporary blues tune, in recognizable temperament, with the flute.[2]
For now, the oldest records of musical instruction with theoretical indication dates to 1500 BC in Akkadian clay tablets.[3] These texts clearly indicate a highly advanced understanding of intervals and music theory that presumably predates the texts, and contain instructions for modulation of strings of an instrument (see picture). From these texts it is possible to interpret a distinct understanding of octave (or unison) interval.

When look at the Akkadian tablets as a historic reference, most of the given information is for tuning in a relative interval system. We can safely assume at this point there are certain melodies or tunes to apply different interval relations. The tuning text is a set of instructions for tuning a Lyre-like stringed instrument known as a sammû. It is a manual for changing the tuning of the instrument from one mode to another, through a cycle of modes. There are 9 strings in this instrument with strings 8 and 9 tuned together with strings 1 and 2, suggesting an understanding of octave or unison interval.
Figure 2. One of two known cuneiform tablets of the tuning text (UET VI/3, 899, British Museum). Photo credit: British Museum
From the translation of the tablet we can conclude that relative pitch system was important in Mesopotamian music, but not a precise pitch. And the tuning was done in order to play certain tunes/melodies.[4]
Perhaps not too dissimilar to the tuning of frets on instruments of Persian traditional music, where the intonation results from the melodic steps.
At the beginning there was melody
Let’s agree that what later formed the foundation of the so-called Western music theory, has its roots in the musical thinking of Greek Antiquity. The theoretical system of ancient Greece, as developed over time by philosophers and mathematicians, did not necessarily and/or immediately corresponded with the practice of music. The practice of Greek music of antiquity, from which very little traces are remained, indicates the existence of different modes. Much like many other old musical cultures, the modes of ancient Greek music were constructed of tetrachords.

In music textbooks Tetrachords are defined as “…modules from which more complex scalar structures may be built”[5]. Perhaps it seems trivial to differentiate musical systems based on tetrachord, after all isn’t a collection of tetrachords automatically creating a scale? We’re not looking at the same thing from 2 angles, we are looking at 2 different musical thinking. What particularly defines scalar music, for me, is a determined destination from which the other steps are divided: an octave interval. Whereas a tetrachord is a rather autonomous unit. In other words, there is no practical linear connection from tetrachord to heptachord or hexachord and octave.
Figure 3. A photograph of the original stone at Delphi containing the second of the two Delphic Hymns to Apollo. The music notation is the line of occasional symbols above the main, uninterrupted line of Greek lettering. Photo credit: Wikipedia creative commons license.
Let’s look at Šur for example, one of the seven Dastgāh of Persian classic music, and arguably the most important one too. The entire Dastgāh could be represented in a sequence of tetrachords that eventually shape a scale. A scale that is not, designed by, following a mathematical formula for its steps/division, but by following its own melodic logic. The tetrachords themselves are not an “interval” that is equally divided into any number of steps either, rather they serve their own purpose: to follow the melody. This scale is just a drawing of all the notes that are at some point played in every Gusheh. Following examples are a grossly simplified and reduced representation of a handful of important Gusheh in this Dastgah.







Figure 4. A transcription of Shur (Šur) and its components. Done by author, from the vocal radif of Mahmoud Karimi.
Gusheh (in plural Gushehā) are considered as the smallest component and building bricks of the Persian classical music. A Gusheh is a small melodic unit, that is collected from tunes or songs of different folkloric and regional-music across Iran. These melodic units which rarely exceed from a tetrachord, have their own intonation and particular melody, sometimes with very specific rhythmic or metric character. These tunes are compiled, assumingly based on their general intonation, into seven Dastgah that form Radif of Persian classical music. In figure 4, we can see that there are accidentals in some of the Gusheh. The factor that decides when to use which intonation/accidental is only the melodic consequences. These melodic figures are being taught and memorized as part of the musical education of Persian classical music. Any attempt to recreate the Persian music from these notation and their intonation without knowing and having heard the exact melodic moves that dictate the intonation, would sound artificial to an Iranian person, even a non-musician one.
Much of the music of Orthodox churches, particularly Greek churches, Persian and Indian music are still based on this principle.
Despite these arguments, there is ample evidence to believe that the octave interval is a defining factor in musical systems of different civilization. And yet the development of musical systems weren’t exactly the same even in light strong harmonic intervals like octave.
Octave and the idea of equal temperament
Even in afore mentioned examples, changing a tetrachord to an octave higher or lower is a common practice. Thusly, octave is not arbitrarily the designated defining interval. Dividing this interval into any arbitrarily selected number of equal steps, however, is a different story.

Figure 5. Bells found at the tomb of Marquis Yi of Zheng. Photo Credt: chinascan.org
The tuning of these semitones is different from the so-called “western” equal temperament, even though these bells resemble 12-tone equal temperament in Pythagorean tuning[7]. The semitones in this ancient Chinese tuning are used as some kind of basis pitch for additional pitches/steps.
It was around 6th century BC when Pythagoras discovered that the frequency of a vibrating string is inversely proportional to its length. And thus he elicited the main intervals according to the harmonics of a string, octave fourth and fifth. This would become the basis for modern tuning systems and equal temperament.
With this understanding alongside the mathematic knowledge already established, the temperament and tuning systems evolved. Most importantly the Pythagorean tuning that is a derivate of a circle of perfect fifths[8]; originally a method to tune the tetrachords, it was adapted to tune a wider range of pitches/scales.
Note that these tuning systems (those based on Pythagorean ratios) coincide with the harmonic relation of intervals, at least in practice. Everything was relatively simple until the development and spreading of keyboard instruments.
The Pythagorean system of tuning was sufficient for the monotonic music of churches and early middle ages. Early and simpler polyphony music could do with some adjustment of thirds.
As the keyboard music becomes more popular and with it the exciting new possibilities of having multiple keys/modes/tones, different issues arise from the old way of just tuning.
Since more and more chromatic pitches were available and used, the discrepancies in Just intonation became more and more evident; always some pitches sounded terribly out of tune and out of context, depending on from which pitch the circle of fifths tuning would begin. And so various compromise attempts appeared to somehow fix or minimize the out-of-tune-ness of just intonation by compromising some lesser used intervals or pitches. Among these attempts are different meantone intonation systems, where a particular interval is slightly compromised in order to keep the other intervals as close to a Just intonation tuning as possible.
These attempts to finding a reasonable balance and compromise in tuning systems eventually concluded in the 12-tone equal system. A tuning that, in the eye of this author, has no regard for the musicality of the intervals but instead finds a perfect mathematical solution for dividing an octave into 12 equally distanced pitches. The solution came in the form of allocating an arbitrary measurement of 100 values as the distance between each semitone of an equally distanced 12-stepped scale, summing up to 1200 value for an entire octave.
The physical understanding of acoustical processes advanced rapidly during and after the Scientific Revolution. Galileo Galilei (1564–1642) independently, discovered the complete laws of vibrating strings (completing what Pythagoras and Pythagoreans had started 2000 years earlier). New knowledge gave a quick and huge impact on redefining the very foundations of music, intonation and instruments; the birth of a new era, paving the way for invention and improvements of keyboard instruments (clavcin and piano) that changed the way the music would be for the centuries to come.
Even complex subjects of acoustic such as interference, reverberation and echoes were first addressed on “De Architectura” of Vitruvius on about 20 BC.
After Galilei and Newton who first used the term “spectrum” in physical science, Joseph Fourier (1768-1830) on his works for solving “heat equation” introduced the series of decomposed periodic functions into the sum of a (possibly infinite) set of simple oscillating functions, later called Fourier Series. This discovery proved to be crucial for a vast spectrum of mathematics, science and engineering, and has many applications among others on electrical engineering, vibration analysis, acoustics, optics, signal processing, image processing, quantum mechanics, econometrics and eventually music.
The eighteenth century saw major advances in acoustics as mathematicians applied the new techniques of calculus to elaborate theories of sound wave propagation. Alongside providing better equipment, perhaps the first major breakthroughs in modern time were works of Joseph Fourier (1768-1830) and later Hermann von Helmholtz (1821-1894).
These discoveries set the grounds for the necessary science behind electronic music and computer music as well as being directly used by composers to create art works; most notably composers of spectral and electronic music.
The advent of spectral music in 20th century saw acoustics becomes a backbone of musical thinking, leading to a boom in related technology and institutions. Such multidisciplinary studies like psychoacoustics are direct results of this boom.
With these arguments, limiting the musical material to 12, or any other number of steps to choose from, seems completely arbitrary and far from the spirit of music through millennia of human civilizations.
Today the studies on sound and acoustics have multiple applications. From telecommunication technologies, radio, to various medical usage and explicit military purposes, to contributions to astronomy and astrophysics.
Collins English Dictionary defines ‘the arts’ as “imaginative, creative, and nonscientific branches of knowledge considered collectively, esp. as studied academically”. This definition may not seem very accurate today anymore.
A personal path
The creative outcome of any artist, scientist, or anyone who creates, lies in a junction between one’s upbringing and childhood, life events and experiences, education, and environment. This collection leads to different levels of awareness on issues such as environmental awareness, historical awareness, sociocultural and political awareness. In my view these are the issues that define an artist’s approach and outcome, and meanwhile make every artist potentially unique on understanding of their surroundings.
Growing up in a small town in highlands of central Iran, far from much influence of western art’s traditions, I was a teenager when first time saw an orchestra on TV. Most of my childhood and early adulthood was spent among telecommunication machinery and equipment of my father’s work place, with their never ending pulses, various beeps and noises that defied any pattern, responsible of all means of communication and connection with the rest of the world. Later as an adult I learned of western classical music and became a pianist and then a contrabassist, as well as playing Persian traditional music and instruments. Listening to Mozart for the first time was as much a life changing experience as playing in Tehran Symphony Orchestra, as in some rare moments there were something very ecstatic in music, something I had never felt before, even as a pianist. There was a magic in some of the pieces, when performed in ensemble/orchestra, that I couldn’t reproduce on piano. It was like a drug!
So I set my goal to discover that magic and how to make it happen; as a composer I wanted to create those ecstatic moments all the time. Only retrospectively I could detect some clues. For instance I figured there is a fundamental difference between western music, and arts, with that of our region’s (middle eastern) art. There seems to be a separation, or distance, in western/European cultures, not only between the artist and the audience but also between the artwork and the audience. The music performance for instance is usually a show, or the music is an object of creating reactions, and being presented to the audience. In eastern, or middle-eastern, traditions, there is no difference between creator and audience and often times it’s hard to distinguish between them. In the case of music more importantly the audience don’t receive the music as a product, but live within it. The audience freely participates in creation of music and the creators are often time anonymous.
The experiencing of the sound from within is a key difference in European traditions and middle-eastern traditions of music. Music is not a tool for expression, but rather music is the life itself. With these realizations, I noticed why I had to invent my way of making music in every step of the way. I could see that my music is following neither eastern nor western norms; I had never learned either of them fundamentally deep enough so that they would’ve shaped my outcome. Instead I learned the sounds from the Morse-code-like noises and pulses of hundreds of machines trying to connect people’s communications to each other, and I had learned the living inside music from impromptus singing of tens of mourners or cheerers in funerals and weddings.
I always thought music and art has to be strong and powerful and so to generate strong reactions, I wanted to recreate the ecstasy that music gave me when first time listened to Mozart and Beethoven. For this I set on a journey to study acoustics and psychoacoustics, to find out what was that feeling of ecstasy, what created it and how to make it happen.
And so as a composer, the material of my music had to be directly sound and acoustical phenomena. I focused the function of my music on the pulsation of energy, which emits through the transformation process of sound itself as a complex unity. Being aware of the power of sound and energy that’s being released/conveyed through interactions and synthesis of sound, I have been interested in taking into account the tiniest interferences that can be perceived to happen between different sounds.
The reason could simply be the fascination that such effects create on myself as a listener, and the amount of energy that are transmitted through simple interactions of sound. It appears to me that interactions between narrow bands of frequency and narrow intervals transmit larger energy than the equal signals of wide intervals.
Meticulous calculations and a good understanding of science, with or without help of technology, make it possible to create the music that was otherwise inconceivable.
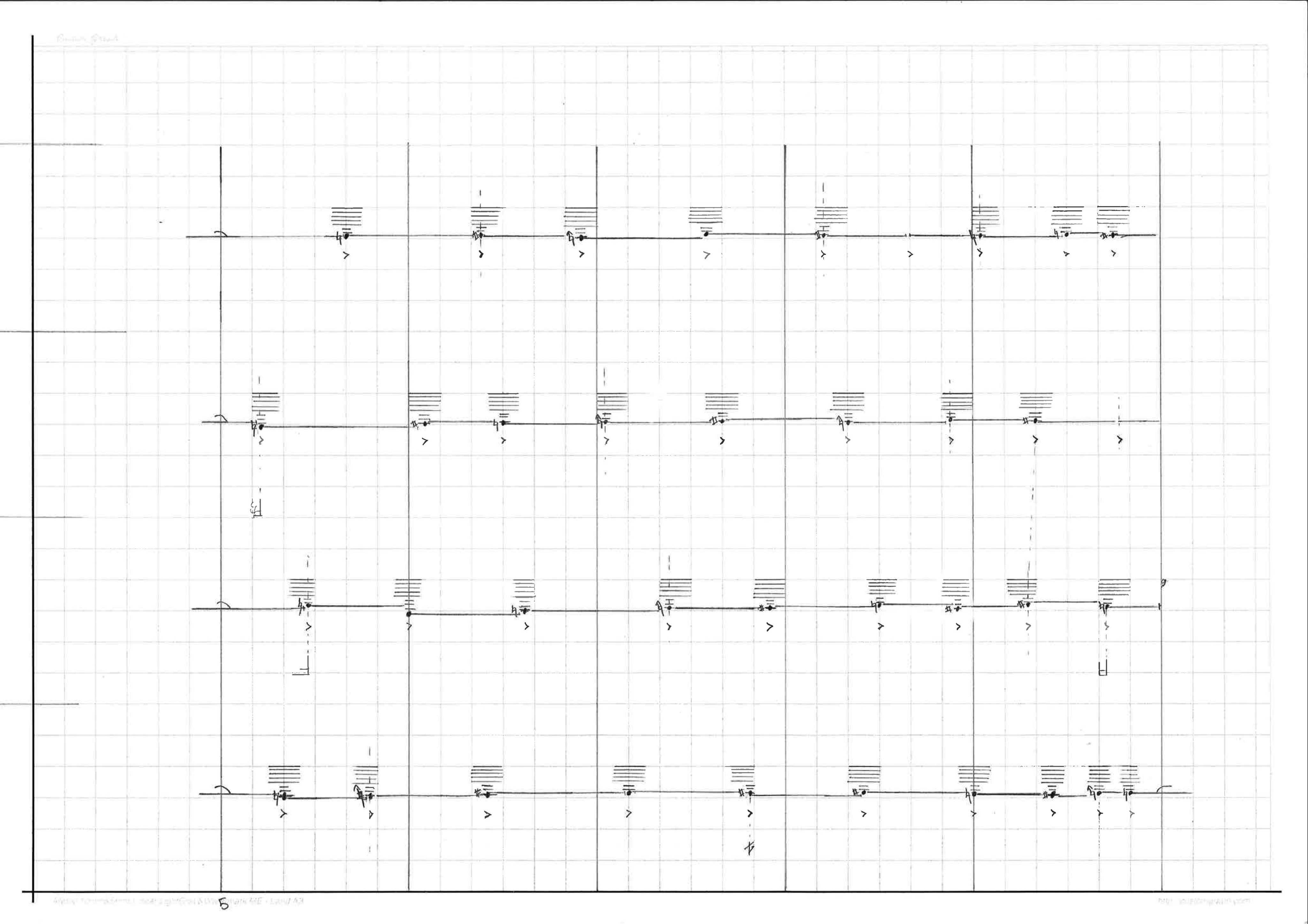
Figure 6. Audio 1. A section from “Finite Functions of infinitive sets”, where simple interferences are used to create calculated beatings.
Two simultaneous simple tones of small frequency difference make interferences that results in periodic variations of amplitude with frequency equal to that of difference between the tones. The amplitude variations could be perceived on as fluctuations on loudness. These loudness fluctuations occur because the two sinusoids interfere on the Basilar Membrane of the inner ear, moving alternately in and out of phase at a rate equal to the frequency difference of the two tones. The fluctuations are referred to as beatings.

When the difference of the two tones is as large as within the range of our hearing (larger than 16Hz), the interference is perceived as an individual tone called combination tone. Combination tones could as well be difference of two initial frequencies (difference tone) and/or the sum of the initial frequencies (summation tone). This phenomenon has been known and practiced as Tartini tone, a difference tone that is still used for fine-tuning certain intervals in string instruments or string/wind chamber ensembles.
Figure 8. Audio 3. Pictures from “Transmittance” where the difference of high frequencies in flute and saxophone are used to create a counter-point melody, here a melody resembling the opening theme of Tristan und Isolde by Wagner is to be heard.
The combination tones are best perceived on interferences of high frequency tones. This may be because of several factors. A mixture of the nonlinear distortions produced in the middle ear and those that are produced in the inner ear might be one reason. Our hearing system shows the best frequency selectivity in a range around 2000 Hz, where the critical bandwidth is relatively small.[9] Interference effects on the BM are complex, as they are influenced by a physiologically active mechanism which introduces strong nonlinearities, including level-dependent amplification.[10]
For a better understanding of how these entities function and affect us, in addition to physics and acoustics one needs to study psychoacoustics. It’s almost inevitable for a composer, working with acoustics, to reach the premises of psychoacoustics. Psychoacoustics is in essence the study of the perception of sound. An interdisciplinary subject between psychology, acoustics, physiology, physics. Psychoacoustics tries to address how we listen, our psychological responses, and the impact of music and sound on our auditory and/or nervous system.
Although the term psychoacoustic itself is relatively new, it is hard to determine when the attention to the psychological side of hearing and perception of sound first raised on science.
On 16th century Galileo wrote “Waves are produced by the vibrations of a sonorous body, which spread through the air, bringing to the tympanum of the ear a stimulus which the mind interprets as sound”, a remarkable statement that points to the beginnings of physiological and psychological acoustics.

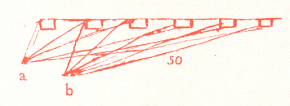
Figure 9. Examples 5-1 and 5-2. Leonardo Da Vinci (1452-1519) representation of a multiple-reflected sound wave and acoustic reflections.
Figure 10. Audio 4. Example of building a harmonic structure entirely upon combination tones, in “Convolutional Emergence”.
In addition to the combination tones, other, maybe less obvious, subjects of acoustics/psychoacoustics studies could be applied in music. Subjects that have been historically, and intuitively, known and practiced by composers to some extent, as basis for instrumentation, orchestration and harmony.


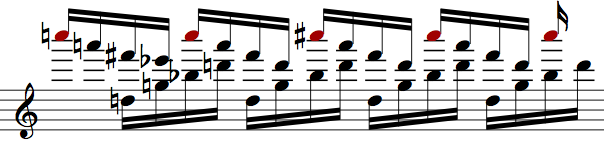

Figure 13. Example of acoustical masking in “Exponential Decay, or there’s nothing to see here”. Compare the scores (left) with the sounding result (right).

Following examples are for a psychoacoustic/acoustic arrangement of repetitive pulses, in a way for them to create a steady and periodic net of beatings. These beatings are meant to create audible sound-waves as counter point with each other and with the played lines by instruments.
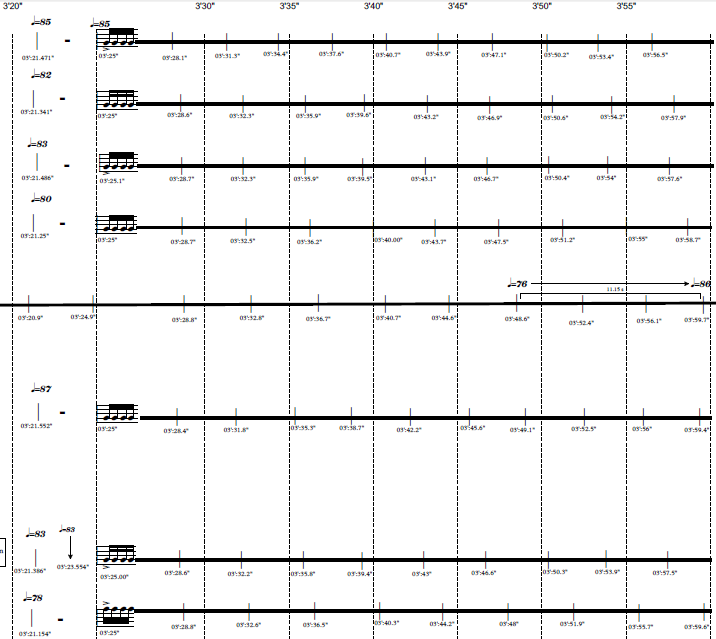
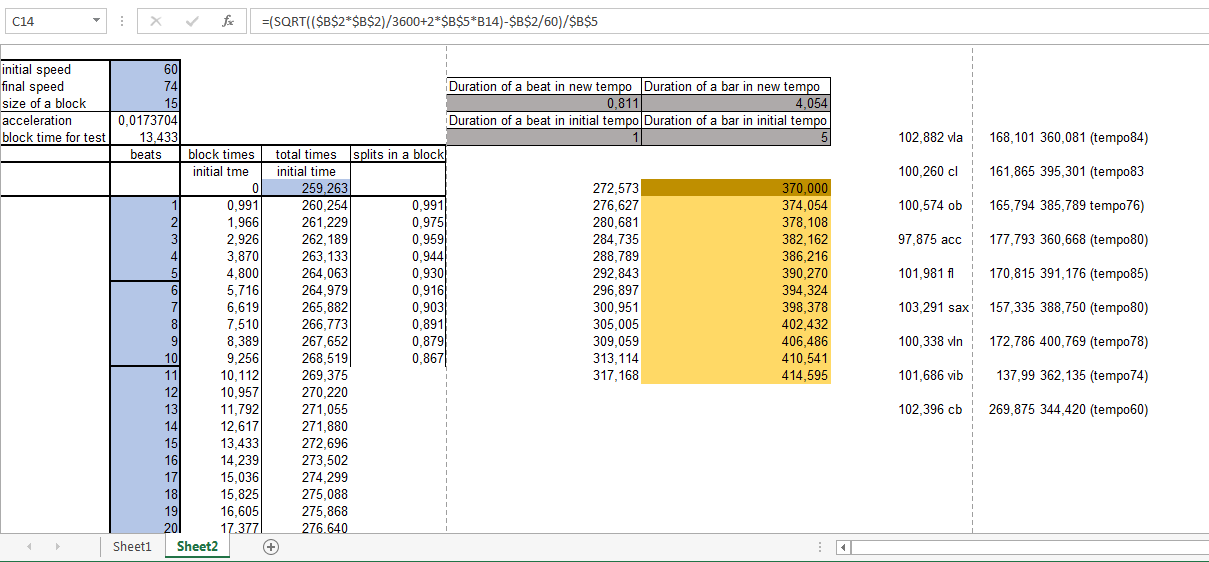
Figure 14. Example of arrangement of pulses in “variations on a theme by R. Beppel”. Note that the timing of each pulse and each barline is calculated as shown in picture below.
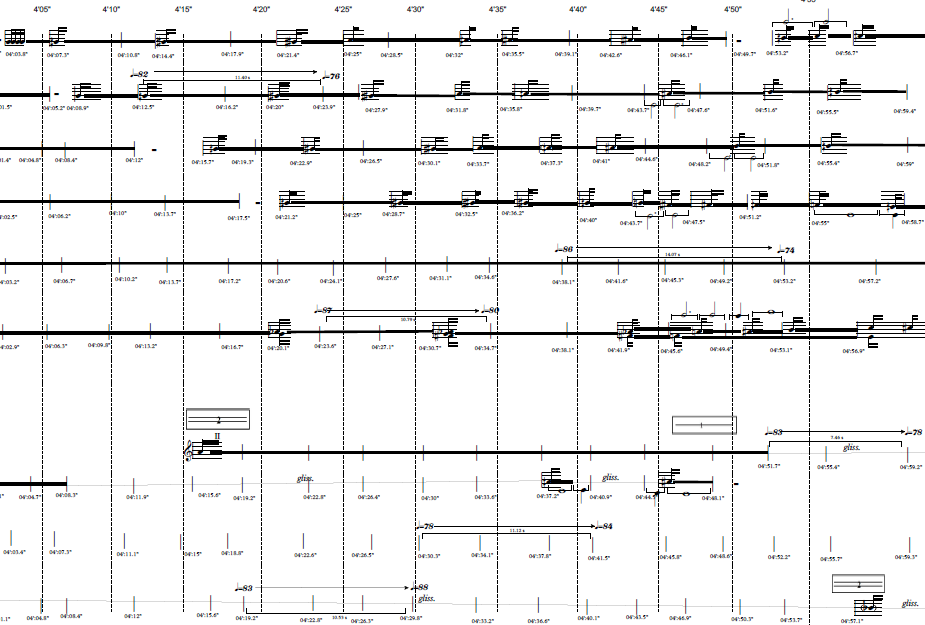
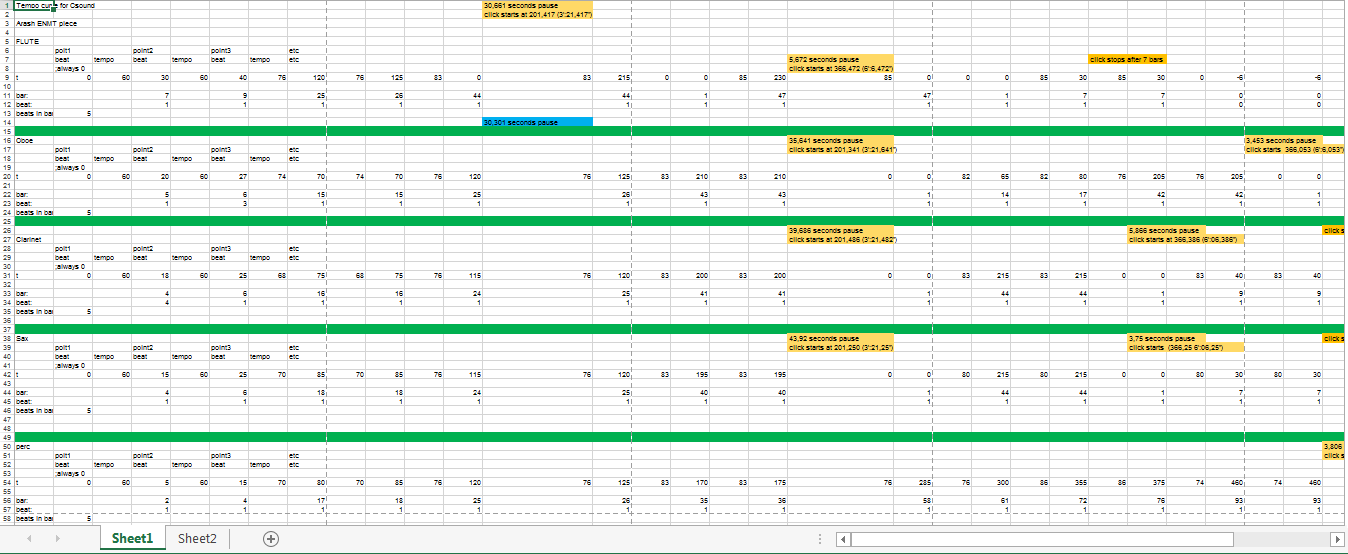
Using a carefully crafted net of acoustical and psychoacoustical phenomena within the layers of music can create a wide range of sensory and auditory experiences. Experiences such as density, tension, pressure, agitation, expectation and sensation of feeling multiple layers of sound.
Figure 17. Audio 6. A complex net of various acoustic and psycho-acoustic (beatings, combination tones, maskings etc.) phenomena in a musical context in Dimension II, Destruction.
Such approach toward music making could be applied to a multitude of layers in composition, from the mechanics of instruments to the very fabric of music. Art supposed to be free and unchained. It supposed to be revolutionary, wild and certainly not tame. And so limiting the creation of music to any pre-determined system is closing some possibilities. I was massively attracted to the idea of microtonal music, as a mean to break free from the rigidness of classical scales and pitch-based music. It was only much later that I realized the accepted definition of “microtonal music” is only used to creating various versions of equally dividing an interval into any arbitrary number of steps[11]. The fascination of such music-making comes primarily from how interesting/peculiar/complicated the ratios/calculations that steps of a scale are derived, could be. And so the microtonal music as is generally accepted has become yet another school of equal temperament.
There are ample historical precedents that practice of art are in defiance of rules or laws of convenience. And yet any kind of knowledge (scientific, cultural, philosophical, political etc.), will enhance and enrich the creation of art. Giving these examples of different musical thinking is an invitation on finding personal and non-conformist ways in music. To question the very fabric of music; even the most universally accepted parameters in music could and should be challenged, for the sole purpose of finding personal musical language.
Bibliography
Sam Mirelman, “Tuning Procedures in Ancient Iraq”, Analytical Approaches to World Music Journal, Vol 2. No 2. 2013
Agnès Garcia-Ventura, Claudia Tavolieri, Lorenzo Verderame, “The Study of Musical Performance in Antiquity”, Archaeology and Written Sources
Eberhard Zwicker and Hugo Fastl, “Psychoacoustics: Facts and Models”, 1990
Brian C. J. Moore, “Interference effects and phase sensitivity in hearing”, 2002
Olson, Harry Ferdinand. Music, Physics and Engineering. New York: Dover Publications 1967.
Boyer C. and Merzbach U. A History of Mathematics. Wiley, 1991.
Anderson, Julian. “A Provisional History of Spectral Music”. Contemporary Music Review 19, no. 2 (200): 7-22. (“Spectral Music: History and Techniques”)
Igari S. “vol II – Fourier Analysis and Integral Transforms”. In “MATHEMATICS: CONCEPTS, AND FOUNDATIONS” in Encyclopedia of Life Support Systems (EOLSS), Developed under the Auspices of the UNESCO, Eolss Publishers, Oxford ,UK.
Railsback, O. L. “Scale Temperament as Applied to Piano Tuning”. The Journal of the Acoustical Society of America 9 (1938): 274.
Austrian Academy of Sciences, Acoustics Research Institute. “Historische Streiflichter zur Psychoakustik”. http://www.kfs.oeaw.ac.at/research/psychoacoustics/history/15_hist.pdf Retrieved April 2014.
“Collins English Dictionary – Complete & Unabridged 11th Edition”. Retrieved October 2014.
- An example being the syntonic comma, that’s been much discussed and argued for centuries, even today; similarly solutions to certain interval ratios that arise specifically from the mathematical problems in Grek antiquity. A recent confusion could be the example of quarter-tone intervals in systems of Persian music, wheras the practice of the music shows application of intervals other than quarter-tone. ↑
- The intonation either is no definitive proof of the flutes tuning, as any wind player knows controlling the intonation is a very challenging practice. ↑
- There are arguably records of musicians life and administrative correspondents from older period, for example in The Ebla Archives, dating back to 3rd millenia BCE. ↑
- For a full translation of the tablet text read: Mirelman, Sam, and Theo J. H Krispijn. 2009. “The Old Babylonian Tuning Text UET VI/3 899.” Iraq 71: 43–52 ↑
- John Cahlmers, “Division of Tetrachords: a prolegomenon to the construction of musical scales”, Frog Peak Music, 1993.↑
- Aristoxenus is believed by some theorists to have firstly thought of equal temperament. ↑
- Shí-èr-lǜ was a standard 12-tone temperament in ancient Chinese music. ↑
- There are signs that Pythagoras took this from Egypt or middle-eastern music and it’s generally believed Babylonian were aware of a practical 12-tone system. ↑
- “Psychoacoustics: Facts and Models” (1990) Eberhard Zwicker and Hugo Fastl ↑
- “Interference effects and phase sensitivity in hearing” (2002) Brian C. J. Moore. ↑
- Or sometimes unequally divided scales. ↑